As promised, I want to now give you some problems to think about, mainly in Chapter 4 and Chapter 5.
But first, a quote for the topologists among us:
It was my lot to plant the harpoon of algebraic topology into the body of the whale of algebraic geometry.
— Solomon Lefschetz (A Page of Mathematical Autobiography, Bulletin of the American Mathematical Society, Volume 74, Number 5, 1968)
The end of Chapter 3
If you are happy with 3.7.C and 3.7.D, then you are happy with the theory of this section. If you can do 3.7.B and 3.7.G, then you can work with these ideas.
The structure sheaf.
Our goal here is to understand the sheaf of functions on (or
) as simply as possible. We want really to know that it is a sheaf, and to be able to work with it. The idea to keep in mind is that we will understand it only through the distinguished open subsets
(where
Doesn’t vanish), and will take the functions on
to be
, except to make sure this is well-defined (i.e, “if
, then
“) we define the functions in a way depending only on the open set itself (Definition 4.1.1).
So Exercise 4.1.A will make sure you are completely comfortable with the second trick. And Exercise 4.1.B and Exercise 4.1.C will make sure you are comfortable with the first.
If you are already familiar with exact sequences, then Remark 4.1.4 will be a clarifying perspective.
The recurring counterexamples in 4.1.6 are good to keep in mind. You might have noticed that an example from pseudolecture 9 was a variant of the last one — is a non-Noetherian scheme for which all the stalks are Noetherian (in fact, they are fields).
Section 4.2 is all about drawing pictures. It should be fun.
In Section 4.3 there are a lot of things to get used to, so a lot of exercises worth doing. You can see if you get confused by 4.3.A. If 4.3.B is straightforward, then you’ll know you are comfortable with these ringed spacdes. 4.3.E(b) is our first (provable) example of a scheme that is not affine!
Exercise 4.3.G will finally make clear that we have defined our locally ringed space with the properties we want.
People often complain that algebraic geometry is sometimes done so “formally” that there are no examples. I agree that it is hard to really understand something without really playing with actual examples. So in 4.4, do what it takes to become comfortable with these three examples. Exercise 4.4.A is one we will use later, for example, when we defined “Proj” in pseudolecture 9. You should do 4.4.D (and then distill it to make it as painless as possible), and then you’ll really know you can compute stuff on a variety, using its cover.
Then in the next section, “Proj” is a machine to make varieties/schemes from many more open sets, but using just one ring. You may want to Exercise 4.5.B to see how homogeneous polynomials “cut out” a scheme in projective space in a hands-on way, so you’ll have a good feel for it when we think about Proj in generality.
Exercise 4.5.D will put graded rings in general into this context. Definitely do Exercise 4.5.E, and take your time with it. (Feel free to solve it in any way you want, even if it involves rearranging some of the development of the material in this section.) The following few exercises fill out the construction of Proj; try a sampling (or all of them!) to convince yourself that there is nothing tricky here. If you prefer compatible germs, do Exercise 4.5.M.
If you have seen these things before, you may want to try 4.5.Q, not because it is fancy, but because it can be confusing, and it relates to a cause of continuing confusion. Grothendieck often thinks of projectivizations of a vector space not as one-dimensional subspaces, but as one-dimensional quotients. So whenever you see “projectivize” in any algebraic geometry paper (or anything very near algebraic geometry), you have to be careful. In fact, there is method behind Grothendieck’s madness (at least this particular madness) — since he is thinking of geometric things in terms of functions on them, he is thinking of the vector space in terms of the linear functions on them (i.e., the dual vector space), and one-dimensional subspaces of a vector spaces indeed correspond to one-dimensional quotients of the dual vector space.
Okay, enough philosophizing! In chapter 5, the first section is just extending topological notions to these new things we have created. The easy exercises are important in that they make sure you can toss these notions around without thinking. I’m somewhat torn about how important 5.1.E really is — but it is worth doing!
I’d like Exercise 5.1.H to be easy, but I fear it is not. Someone should try it and let me know!
In section 5.2, the key exercise to do is Exercise 5.2.F (integral = reduced + irreducible). Exercise 5.2.H involves an important concept as well. Exercise 5.2.I is why some people start with irreducible varieties, in order to make the sheafy issues less scary.
Section 5.3 is home to the Affine Communication Lemma, which we will use repeatedly, and with great effect. You should understand the proof, and why there isn’t much there! Then the exercises give you lots of opportunities to practice with it. You should try some of the exercises involving the new notions (such as Noetherianness of schemes), but the ones I’ll most suggest you do is 5.3.H and 5.3.I.
Logically (and in pseudolecture 9), section 5.4 could come after 5.2, as it involves more “stalk-local” properties. There are some exercises to make sure you see how the theory fits together (such as 5.4.F), but the really fun ones deal with actual explicit rings (5.4.G to 5.4.L; 5.H is much more useful than it looks).
Then skip the rest of Chapter 5 (I’m in the process of completely rewriting the part on associated points and associated primes), and we will discuss morphisms of schemes in pseudolecture 10!
Plan for these problem.
You can think about these problems for the next couple of weeks. But if by next Wednesday (September 2) you can send your shepherd (or indeed any shepherd) an update on (a) what you have been working on, and (b) how it is going, that will help me figure out what to say next. Also please tell them (c) what is the most confusing thing, (d) what is the coolest thing, (e) what exercise you most want to see an answer to, and (f) what exercise you liked best.
And lastly, more inspired by the question of why the sheaf of functions is called , from Tomas Prochazka.
Hi Ravi,
I think you mentioned the linguistic origin of O? I read last year something about the origin of some notation in math (I don’t know to what extent is it reliable but it kind of makes sense):
O … holomorphic from Italian (where they don’t use H, not even in this word) 🙂 it’s quite funny if it is true
k … for field from German Körper (body, that’s how field is called I think in some languages, including Czech)
Z … for integers, in German Zahlen – numbers
e … for unit, again German from einheit
U,V … for open sets, German and French Umgebung (neighbourhood) and voisinage (I don’t know French)
F,G … in topology, ferme, Gebiet
finally they also said that Klein bottle was not a bottle but a surface (in German Flasche vs Fläche) but somehow there was a typo or misunderstanding and they started translating it as a bottle.
August 27, 2020 at 9:07 pm
The 1-d subspaces vs. quotients really look different if one tries projectivizing ZZ^n. There are way more injections ZZ -> ZZ^n than surjections ZZ^n ->> ZZ.
August 29, 2020 at 7:16 pm
Only by a factor of zeta(n) !
August 30, 2020 at 7:35 pm
(Touche, David!) Allen, I suspect you are intending to show a lot with that example, and perhaps want to say more?.
I’ll point out to readers that the surjections all have kernels that are free
all have kernels that are free 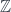 -modules, while the injections don’t all have cokernels that are free
-modules, while the injections don’t all have cokernels that are free 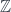 -modules.
-modules.